Adjoint Representation

Prerequisites: Representation Theory, Lie Algebras. Full path (5 pages)

• Intuitive
• Concrete
• Abstract
• Why is it interesting?

Intuitive

The adjoint representation describes how the generators of the group, which live in the Lie algebra, transform. In other words, the adjoint representation acts on the Lie algebra. Note that the Lie algebra is a regular vector space and thus can serve as a representation space.

The dimensionality of the adjoint representation is equal to that of the Lie algebra. For example, the adjoint representation of $SU(2)$ is 3 dimensional and the adjoint representation of $SU(3)$ is 8 dimensional.

An important application of the adjoint representation is the transformation of gauge fields.

Concrete

• For a detailed discussion, see What’s so special about the adjoint representation of a Lie group? by J. Schwichtenberg

Example

The diagram below shows the defining representation of $SU(2)$ in its upper branch. To construct the adjoint representation, we use the Lie algebra as the representation space, as shown in the lower branch (red arrows). The group elements act on this space like $L'=ULU^{-1}$ and the Lie-algebra elements act like $L'=[J,L]$. It is possible to rewrite the adjoint representation such that it acts on 3-dimensional vectors (as opposed to 2x2 matrices) by regular matrix-vector multiplication.

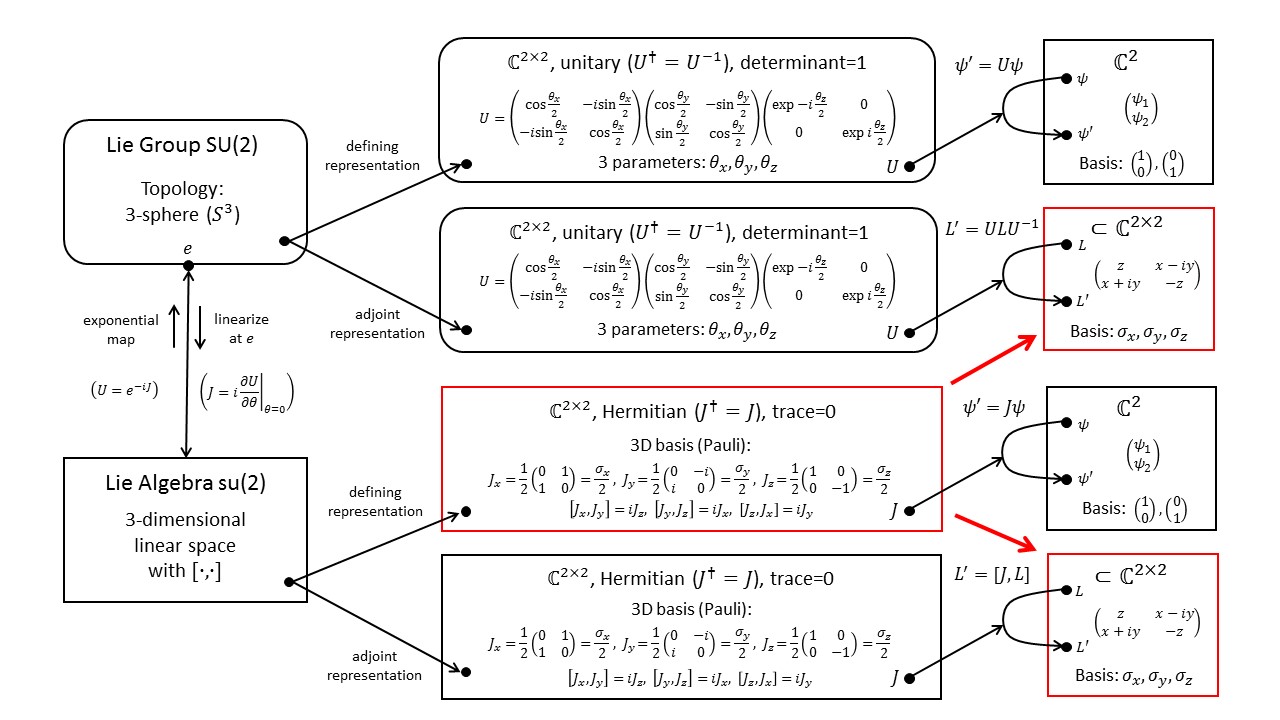
For a more detailed explanation of this diagram as well as adjoint representations of other groups, see Fun with Symmetry.

Abstract

Let $G$ be a Lie group with Lie algebra $\mathfrak{g}$. Conjugation $c_g(h) = ghg^{-1}$ fixes the identity, so its derivative there is a linear map of $\mathfrak{g} = T_eG$ to itself: $$\mathrm{Ad}_g(X) = \frac{d}{dt}\Big|_{t=0}\, g\,\mathrm{e}^{tX}g^{-1},$$ which for matrix groups is $\mathrm{Ad}_g(X) = gXg^{-1}$. The map $\mathrm{Ad}: G\to GL(\mathfrak{g})$ is the adjoint representation of the group. Its derivative at the identity is the adjoint representation of the algebra, $$\mathrm{ad}_X(Y) = [X,Y], \qquad \mathrm{Ad}_{\mathrm{e}^X} = \mathrm{e}^{\mathrm{ad}_X} .$$ That $\mathrm{ad}$ is a Lie algebra homomorphism, $\mathrm{ad}_{[X,Y]} = [\mathrm{ad}_X,\mathrm{ad}_Y]$, is exactly the Jacobi identity. In a basis, $(\mathrm{ad}_{X_a})^c{}_b = c^c{}_{ab}$: the structure constants are the matrices of the adjoint representation.

Kernel and image. For connected $G$ the kernel of $\mathrm{Ad}$ is the centre $Z(G)$, so $\mathrm{Ad}(G)\cong G/Z(G)$. For $SU(2)$ this is the double cover $SU(2)\to SO(3)$; for $SU(N)$ the image is $SU(N)/\mathbb{Z}_N$. The kernel of $\mathrm{ad}$ is the centre of $\mathfrak{g}$, which vanishes for semisimple algebras, so $\mathrm{ad}$ is then faithful. The adjoint representation of a semisimple algebra is irreducible exactly when the algebra is simple.

Roots and invariants. For complex semisimple $\mathfrak{g}$ with Cartan subalgebra $\mathfrak{h}$, the non-zero weights of the adjoint representation are the roots, and $\mathfrak{g} = \mathfrak{h}\oplus\bigoplus_\alpha\mathfrak{g}_\alpha$ is the root space decomposition. The highest weight of the adjoint representation of a simple algebra is the highest root. For $\mathfrak{su}(3)$ the eight dimensions consist of six roots and the two-dimensional Cartan subalgebra. The Killing form $B(X,Y)=\mathrm{tr}(\mathrm{ad}_X\mathrm{ad}_Y)$ is $\mathrm{Ad}$-invariant, $B(\mathrm{Ad}_gX,\mathrm{Ad}_gY) = B(X,Y)$, and for semisimple $\mathfrak{g}$ it identifies the adjoint representation with the coadjoint representation on $\mathfrak{g}^*$. Coadjoint orbits carry a natural symplectic structure (Kirillov-Kostant-Souriau), and their quantisation is one route to the irreducible representations.

Gauge theory. A connection on a principal $G$-bundle is locally a $\mathfrak{g}$-valued one-form $A$. With covariant derivative $D = d + A$ on a field in a representation $\rho$, a gauge transformation acts as $$A\mapsto gAg^{-1} + g\,d(g^{-1}), \qquad F = dA + A\wedge A\mapsto gFg^{-1},$$ where $g$ and $A$ are understood in the representation $\rho$. The curvature $F$ transforms in the adjoint representation, while $A$ transforms in it only up to the inhomogeneous term. The gauge bosons therefore come in the adjoint representation, and their number equals $\dim G$, for example eight gluons for $SU(3)$.

Why is it interesting?

A representation is a map that maps each element of the set of abstract groups element to a matrix that acts on a vector space. A confusing point here is: If we can study the representation of any group on any vector space, where should we start?

Luckily there comes exactly only distinguished vector space automatically with each Lie group: the Lie algebra of the group!

The representation of each group where it acts on its own Lie algebra is called the adjoint representation.

Contributing authors:

Path to this page

1. Linear Algebra
2. Group Theory
3. Lie Groups
4. Representation Theory
5. Lie Algebras

Authors: Ares Marrero, Eduard Sackinger