Intuitive

The Klein-Gordon equation describes how the state of a relativistic (= fast moving) quantum system without spin changes in time.

The analogous equation for systems with half-integer spin is the Dirac Equation.

If the system only moves slowly, the Klein-Gordon equation becomes the Schrödinger Equation.

Concrete

The Klein-Gordon equation can be derived from the Lagrangian

\begin{equation} \mathscr{L}= \frac{1}{2}( \partial _{\mu} \Phi \partial ^{\mu} \Phi -m^2 \Phi^2) \end{equation}

using the Euler-Lagrange equations.

Solutions

The most general solution of the Klein-Gordon equation is\begin{equation}\label{KGsol} \Phi(x)= \int \mathrm{d }k^3 \frac{1}{(2\pi)^3 2\omega_k} \left( a(k){\mathrm{e }}^{ -i(k x)} + a^\dagger(k) {\mathrm{e }}^{ i(kx)}\right) .\end{equation}

• A nice discussion can be found in chapter 3 of Klauber's Student Friendly QFT book
• For an elementary derivation of the Klein-Gordon equation see Physics from Symmetry by Schwichtenberg

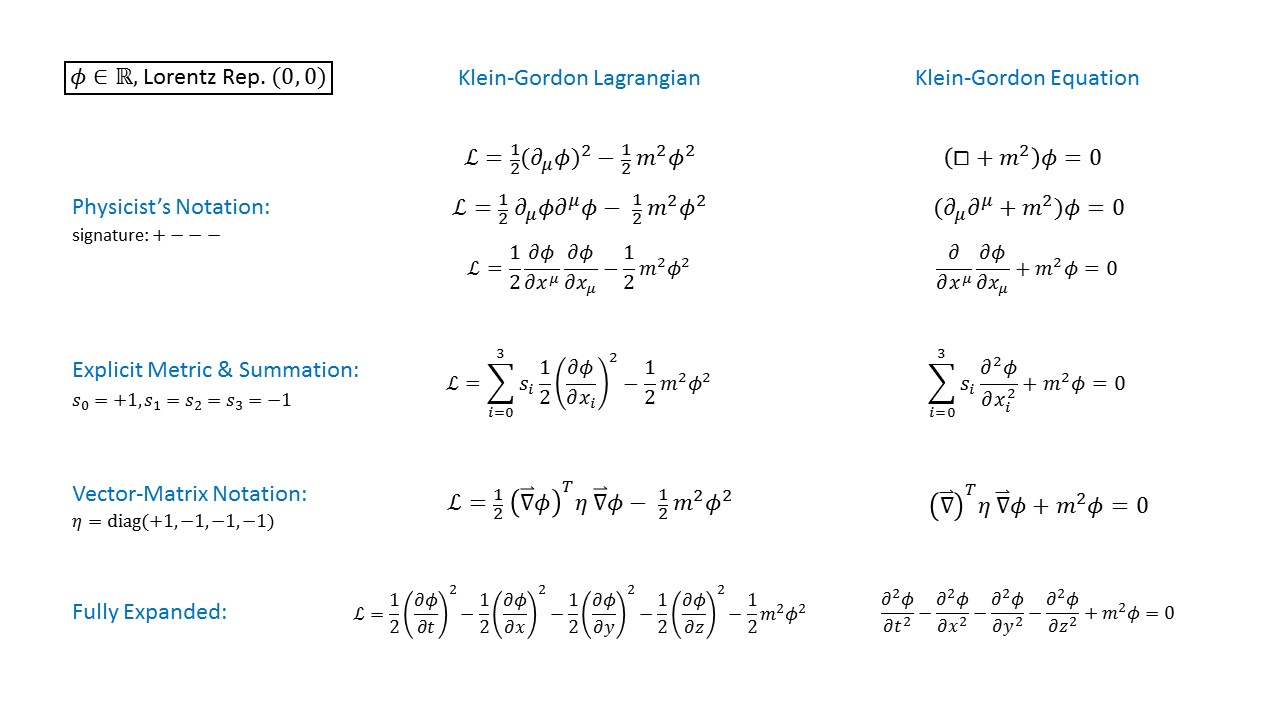
Graphical Summary

The diagram below shows the Klein-Gordon equation and its Lagrangian in various forms. For a more detailed explanation see Fun with Symmetry.

Abstract

Why is it interesting?

The Klein-Gordon equation is the correct equation of motion that describes free spin $0$ particles. For a spin-1 generalization see the Duffin-Kemmer-Petiau equation.

Definitions

• $\partial _{\mu} $ denotes the partial derivative and $\partial _{\mu} \partial ^{\mu}$ stands for a sum using the Einstein sum convention, i.e. $\partial _{\mu} \partial ^{\mu} = \partial _0 \partial^0 - \partial _1 \partial^1 -\partial _2 \partial^2 -\partial _3 \partial^3$,
• $m$ denotes the mass of the particle,
• $\Phi$ describes the spin $0$ field if we work in a field theory.
• Note: $\Phi$ cannot be interpreted as a wavefunction because it is a real valued field; it is its own anti-particle like the Majorana fermion. Only in the case that it is the U(1)-charged (requires 2 independent real Klein Gordon fields that are symmetry transform into each other) is a naive wavefunction interpretation possible. Basically, you get a relativistic scalar superfluid field. Nevertheless, there are single particle wavefunctions lurking in the single real Klein-Gordon theory. But you need to use the coherent state representation to see the 1st quantized operators from the complex annihilation and creation operators. Essentially undoing the second quantization.