Intuitive

Concrete

In Lagrangian mechanics we define a quantity \begin{equation} L\equiv K(t)-V(q(t)) \end{equation} called the Lagrangian. In addition, for any trajectory $q \colon [t_0,t_1]\rightarrow\mathbb{R}^n$ with $q(t_0)=a$, $q(t_1)=b$, we we define the corresponding action \begin{equation} S(q)\equiv \int_{t_0}^{t_1}L(t)\,dt. \end{equation}

Source: Lectures on Classical Mechanics by John C. Baez

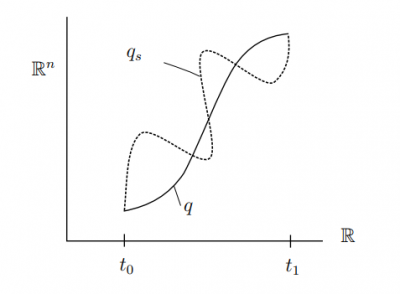
The basic idea is now that nature causes particles to follow the trajectories with the least amount of action.

Using this approach, we can derive Newtonian mechanics. Alternatively, we can start with Newtonian mechanics and derive Lagrangian mechanics.

Abstract

Lagrangian mechanics can be formulated geometrically using fibre bundles.

The Lagrangian function is defined on the tangent bundle $T(C)$ of the configuration space $C$.

In contrast, the Hamiltonian function is defined on the cotangent bundle $T^\star(C)$, which is also called phase space.

The map from $T^\star(C) \leftrightarrow T(C)$ is called Legendre transformation.

• See page 471 in Road to Reality by R. Penrose, page 167 in Geometric Methods of Mathematical Physics by B. Schutz and http://philsci-archive.pitt.edu/2362/1/Part1ButterfForBub.pdf.

Why is it interesting?