Exterior Derivative

Prerequisites: Differential Forms. Full path (5 pages)

• Intuitive
• Concrete
• Abstract

Intuitive

The exterior derivative generalizes the curl operator from 3-dimensional space to any number of dimensions.

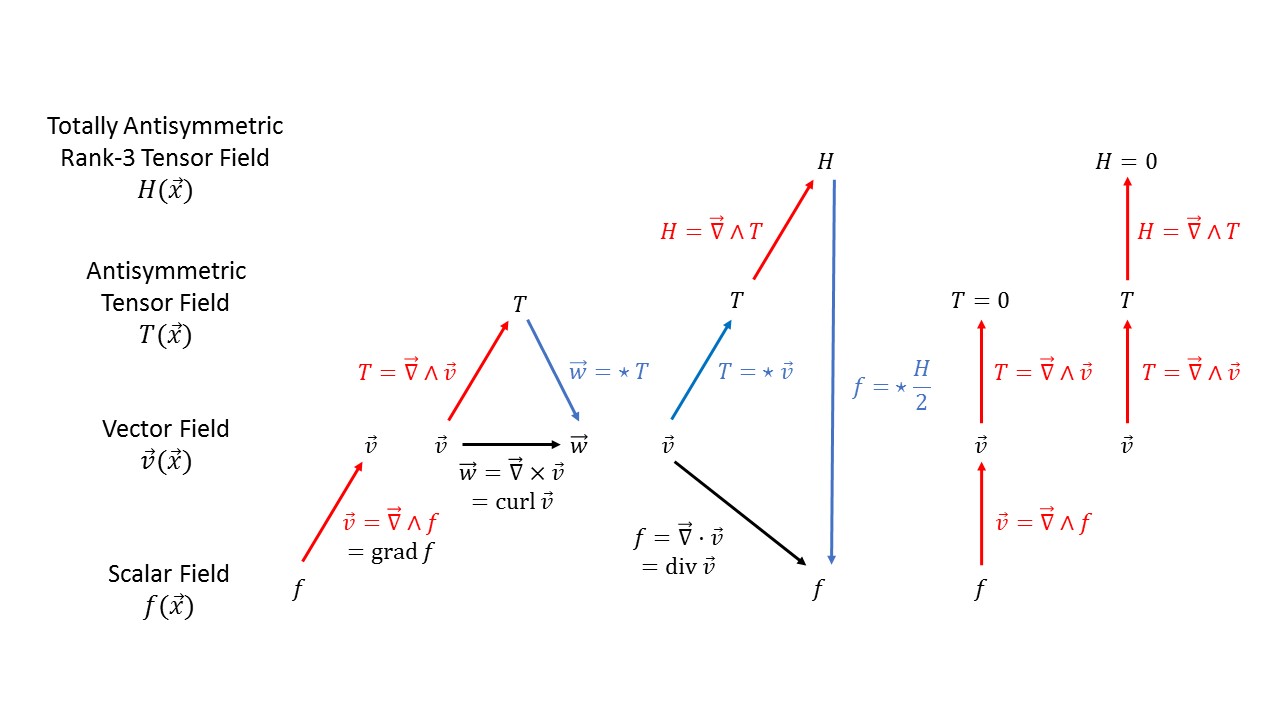
The exterior derivative of a vector field $v$ can be written as $\nabla \wedge v$, where the $\wedge$ indicates the exterior product. This is analogous to how we can write the curl as $\nabla \times v$, where $\times$ is the cross product, and the divergence as $\nabla \cdot v$, where $\cdot$ is the dot product. The result of $\nabla \wedge v$ is an anti-symmetric tensor field.

In 3-dimensional space, the curl operator is equal to the Hodge dual of the exterior derivative: ${\rm curl}(v) = \star(\nabla \wedge v)$.

The exterior derivative of a $p$-form $\omega$ is commonly written as $d\omega$. The result of $d\omega$ is a ($p+1$)-form.

Taking the exterior derivative twice (of any object) results in zero: $d^2\omega=0$. This is an important result with many implications for electrodynamics, topology, etc.

Concrete

The diagram shows on the left-hand side how the gradient, curl, and divergence operators know from 3D can be constructed from the exterior derivative and the Hodge dual. The right-hand side illustrates the fact that taking the exterior derivative twice results in zero.

For a more detailed explanation of this picture see Fun with Symmetry.

Abstract

Let $M$ be a smooth $n$-manifold and $\Omega^k(M)$ the space of smooth $k$-forms, the sections of $\Lambda^kT^*M$. The exterior derivative is the unique family of $\mathbb{R}$-linear maps $d:\Omega^k(M)\to\Omega^{k+1}(M)$ such that

• on functions $df$ is the differential, $df(X)=X(f)$,
• $d\circ d=0$,
• $d(\alpha\wedge\beta)=d\alpha\wedge\beta+(-1)^k\alpha\wedge d\beta$ for $\alpha\in\Omega^k(M)$ (graded Leibniz rule).

In local coordinates, for $\omega=\frac{1}{k!}\,\omega_{i_1\cdots i_k}\,dx^{i_1}\wedge\cdots\wedge dx^{i_k}$ with antisymmetric components, $$d\omega=\frac{1}{k!}\,\partial_j\omega_{i_1\cdots i_k}\,dx^j\wedge dx^{i_1}\wedge\cdots\wedge dx^{i_k}.$$ The property $d^2=0$ follows from the symmetry of second partial derivatives, $\partial_i\partial_j=\partial_j\partial_i$, contracted with the antisymmetric $dx^i\wedge dx^j$. No connection or metric is needed: $d$ is defined on any smooth manifold. A coordinate-free formula for vector fields $X_0,\dots,X_k$ is $$d\omega(X_0,\dots,X_k)=\sum_i(-1)^iX_i\big(\omega(\dots,\hat X_i,\dots)\big)+\sum_{i<j}(-1)^{i+j}\omega([X_i,X_j],\dots,\hat X_i,\dots,\hat X_j,\dots),$$ where a hat means the argument is omitted (this uses the convention in which $dx\wedge dy=dx\otimes dy-dy\otimes dx$).

Structural properties.

• Naturality: for a smooth map $f:M\to N$, $d(f^*\omega)=f^*(d\omega)$.
• Cartan's formula for the Lie derivative: $\mathcal{L}_X=d\,\iota_X+\iota_X\,d$, where $\iota_X$ is contraction with $X$.
• Stokes' theorem: for a compact oriented manifold with boundary and $\omega\in\Omega^{n-1}(M)$, $\int_Md\omega=\int_{\partial M}\omega$. This contains the fundamental theorem of calculus and the theorems of Gauss, Green and Stokes of vector calculus.

Cohomology. Since $d^2=0$, $(\Omega^\bullet(M),d)$ is a cochain complex, the de Rham complex. Its cohomology $H^k_{dR}(M)=\ker d|_{\Omega^k}/\operatorname{im}d|_{\Omega^{k-1}}$ measures closed forms that are not exact. The Poincaré lemma states that on a contractible open set every closed form is exact. De Rham's theorem identifies $H^k_{dR}(M)$ with the singular cohomology $H^k(M;\mathbb{R})$, so the failure of closed forms to be exact detects the topology of $M$.

Physics. In electrodynamics the field strength is a 2-form $F=dA$ with gauge potential $A$. The homogeneous Maxwell equations are $dF=0$, which holds automatically because $d^2=0$, and the gauge transformation $A\to A+d\lambda$ leaves $F$ unchanged for the same reason. The inhomogeneous equations take the form $d\star F=\star J$ up to a sign that depends on conventions. On a space with nontrivial $H^2$, such as $\mathbb{R}^3$ minus a point, a closed $F$ need not be globally exact, which is the setting of the Dirac monopole.

Contributing authors:

Path to this page

1. Calculus
2. Linear Algebra
3. Manifold
4. Vector Calculus
5. Differential Forms

Authors: Eduard Sackinger