Why is it interesting?

Layman

Student

Illustration of the map from some two-dimensional manifold $M$ onto $R^2$. The neighborhood $U$ of $P$ in $M$ is mapped onto $V$ in $R^2$. This map provides a coordinate system in the neighborhood of $P$.

The standard sentence is: A Manifold is a set of points that locally "looks the same" as some Euclidean space. Such geometric spaces are particularly nice to investigate, because we can use, locally, the familiar tools from elementary calculus. Happily, such spaces are not only nice to investigate, but also useful in physics to describe nature.

The idea of locality is made precise by the mathematical concept of a neighborhood. To each point of the manifold, a neighborhood must exist that is just like a small piece of Euclidean space.

Illustration of the map from some two-dimensional manifold $M$ onto $R^2$. The neighborhood $U$ of $P$ in $M$ is mapped onto $V$ in $R^2$. This map provides a coordinate system in the neighborhood of $P$. The idea of "looks like Euclidean space" is made precise by the concept of a diffeomorphism. If there exists a map: smooth, one-to-one, onto, and with a smooth inverse, (=a diffeomorphism) for each neighborhood onto some piece of Euclidean space, the space in question is a manifold.

This map from some neighborhood $X$ of the manifold onto Euclidean space gives us local coordinates of the points in this neighborhood. The inverse map is called a parametrization of the neighborhood. Therefore another way of thinking about an n-dimensional manifold is that its a set which can be given n independent coordinates ins some neighborhood of any point.

Researcher

Examples

The Unit Circle

Image by derivative work: Pbroks13 (talk)Circle_with_overlapping_manifold_charts.png: KSmrq (Circle_with_overlapping_manifold_charts.png) [CC-BY-SA-3.0 (http://creativecommons.org/licenses/by-sa/3.0)], via Wikimedia Commons

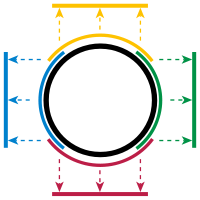
An example for a manifold is the unit circle $S^1$, mathematically defined as the set of points fulfilling the condition $x^2+y^2=1$. This is commomly expressed as:

\begin{equation} S^1 = \{ (x,y) \in R^2 : x^2+y^2 =1 \} \end{equation}

This manifold is one dimensional, hence the $1$ behind the $S$, because two degrees of freedom $x,y$ are reduced to one by the condition $x^2+y^2=1$. To see that this is a manifold, we need to find a map to each neighborhood onto Euclidean space.

The upper semicircle ($y>0$) is parametrized by $\Phi_1(x)=(x,\sqrt{1-x^2})$. $\Phi_1(x)$ maps the open interval $-1<x<1$ bijectively onto the upper semicircle. The inverse map is smooth and gives us coordinates of the upper semicircle.

For the lower semicircle ($y<0$) a parametrization is $\Phi_2(x)= (x,-\sqrt{1-x^2})$. Therefore we have found so far two local parametrizations.

These maps do not cover the points $(1,0)$ and $(-1,0)$ and we need further maps for the neighborhoods of these points: $\Phi_3(y)= (\sqrt{1-y^2},y)$ and $\Phi_4(y)= (-\sqrt{1-y^2},y)$. These maps are appropriate for the right- and left-half the circle. Nevertheless they not include $(0,1)$ and $(0,-1)$.

We can see that the neighborhoods overlap, which is no problem.

FAQ

History