String Theory

Intuitive

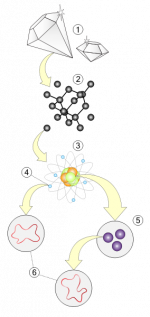
Image by MissMJ published under the CC BY 3.0 licence

The basic idea of string theory is that all fundamental particles are not really pointlike, but rather tiny vibrating strings. We only perceive them as pointlike because we are with our current technology too far away to see that they are actually string.

Different particles correspond to different vibration modes. In this sense the hope is to understand the observed spectrum of elementary particles.

Legend for the image on the right-hand side:

  1. Matter
  2. Molecular structure (atoms)
  3. Atom (protons, neutrons, electrons)
  4. Electron
  5. Quarks
  6. Strings

Recommended Layman Resources:

Concrete

The best introductory textbooks are:

  • A First Course in String Theory by B. Zwiebach
  • String Theory in a Nutshell by E. Kiritsis
  • Lectures on String Theory by D. Tong

The standard textbooks are:

  • Gauge Fields and Strings by Polyakov
  • Superstring theory by Green, Schwarz, Witten
  • String Theory and M-Theory: A Modern Introduction by Becker, Becker, Schwarz

Further Resources

Abstract

The motto in this section is: the higher the level of abstraction, the better.

Why is it interesting?

String theory, for vacua with non-compact dimensions, ‘predicts’ gravity and at least one neutral scalar, the dilaton, antisymmetric tensors of different ranks and usually also charged matter, and supersymmetry (see for instance [1]). A Comment on Continuous Spin Representations of the Poincar´e Group and Perturbative String Theory by Anamarıa Font et. al.

See also:

Criticism

Source: https://xkcd.com/171/

„String theorists hoped to uncover a theory of everything that would contain both the standard model and general relativity. But starting in the late 1980s, it became increasingly clear that the theory cannot predict which particles, fields, and parameters we have in the standard model. Instead, string theory gives rise to a whole landscape of possibilities. In this landscape every point corresponds to a different version of the theory with different particles and different parameters and different laws of nature. If one believes that string theory is the final theory, then this lack of predictability is a big problem: it means the theory cannot explain why we observe this particular universe. Hence, to make the final theory claim consistent with the lack of predictability, string theorists had to accept that any possible universe in the landscape has the same right to existence as ours. Consequently, we live in a multiverse. The string theory landscape conveniently merged with eternal inf“ Lost in Math by Sabine Hossenfelder

I do feel strongly that this is nonsense! … So perhaps I could entertain future historians by saying I think all this superstring stuff is crazy and is in the wrong direction. … I don’t like it that they’re not calculating anything. … why are the masses of the various particles such as quarks what they are? All these numbers … have no explanations in these string theories – absolutely none! … I don’t like that they don’t check their ideas. I don’t like that for anything that disagrees with an experiment, they cook up an explanation—a fix-up to say, “Well, it might be true.” For example, the theory requires ten dimensions. Well, maybe there’s a way of wrapping up six of the dimensions. Yes, that’s all possible mathematically, but why not seven? When they write their equation, the equation should decide how many of these things get wrapped up, not the desire to agree with experiment. In other words, there’s no reason whatsoever in superstring theory that it isn’t eight out of the ten dimensions that get wrapped up and that the result is only two dimensions, which would be completely in disagreement with experience. So the fact that it might disagree with experience is very tenuous, it doesn’t produce anything. Richard Feynman in an interview published in Superstrings: A Theory of Everything? (1988) edited by Paul C. W. Davies and Julian R. Brown,

“In my opinion, string theory in general may be too ambitious. We know too little about string dynamics to attack the fundamental questions of the ‘right’ vacua, hierarchies, to choose between anthropic and misanthropic principles, etc. The lack of control from the experiment makes going astray almost inevitable. I hope that gauge/string duality somewhat improves the situation. There we do have some control, both from experiment and from numerical simulations. Perhaps it will help to restore the mental health of string theory.”Confinement and Liberation by A. M. Polyakov

The need to accommodate handedness in the weak interaction thus becomes an acid test of all putative theories of everything. The Application of this test to superstring theory produced a remarkable result. The original twenty-six-dimensional string theory was unique: the string was a featureless line wiggling around in twenty-six-dimensional space. But the transformation of the twenty-six dimensional string into the ten-dimensional string with supersymmetry is far from unique; the sixteen dimensions rolled up in the internal structure of the string can be rolled up in an enormous number of ways. This is a matter of straightforward geometry, just as it was for the rolling up of the extra dimensions of eleven dimensional supergravity theories to get the required four-dimensional world. If the aim is to find a unique theory of everything, in the form of a special ten-dimensional string theory preferred to all others, this abundance of possibilities does not look promising. But at this point came the second great advance of 1984. Of all the possible ten-dimensional string theories. Green and Schwarz Were able to find only one that could accommodate handedness in the interactions without breaking down. This seemingly unique theory was given the cryptic designation SO(32), which arose from the mathematicians' enumeration scheme for all the geometrical ways of rolling up sixteen of the twenty-six dimensions of the original string to get a ten-dimensional superstring. For the happy result that out of all the mathematically possible super string theories only one had the capacity for handedness, physi cists could only thank, once again, the gods of mathematics. It Was a striking faa, all the more striking for being quite inexplicable. There was no reason why a theory of formerly twenty-six dimensional strings wrapped into ten dimensions and incorporating supersymmetry in a certain specific way should turn out to be the only theory possible. The whole thing was a gift to physics from the world of pure mathematics, an answer to Einstein's Desire to know whether "God had any choice in the creation ofthe world." The answer, it seemed, was. No: God had to use theSO(32) string in ten dimensions, just like the rest of us. This moment of perfea fulfillment was sadly brief. A little later,a second string theory, the equally cryptic Eg x Eg, was also found to be able to accommodate handedness, thereby passing the same acid test. There was a story going around physics departments at the time that Richard Feynman had been quite enamored of string theory when he heard about Schwarz's and Green's result,but that when he heard that two such theories were possible he consigned the whole enterprise to the growing junk heap of would-be theories of everything. Despite Feynman's skepticism,however, string theories have not proliferated. For most physicists it remains an astonishing fact, impossible to ignore, that an elementary application of geometrical laws should selea only two theories from the multitude of possibilities. When so remarkable a result comes with such unanticipated force into the minds of theoretical physicists, must we not accept that something out of the ordinary has been discovered? How can superstring theory not be the foundation for a theory of everything? At this point, however, the difficulties of the "top-down"approach to doing physics can no longer be skirted. We should review briefly what has brought us to this juncture in theoretical physics. The demi-uniqueness of string theories arises from the application of general principles: any string theory is free of infinities of the sort that have to be "renormalized" away in quantum electrodynamics and the other point-particle quantum theories; of string theories, only the twenty-six-dimensional one is free of faster-han-light phenomena; this theory contains only bosons,but can be reduced to a ten-dimensional superstring theory that contains both fermions and bosons, related by supersymmetry;this reduction can be be done in an enormous number of ways>but of all these only the SO(32) and Eg x Eg ten-dimensional strings successfully accommodate interactions with handedness.Finally, superstring theories contain bosons with two units of spin, which can be identified with gravitons, allowing the possibility of a connection to a quantum theory of gravity and thence to a classical theory of gravity resembling general relativity. These are all desirable and perhaps essential characteristics, qualities that any theory of everything must, at a minimum, possess. But where's the real physics in this? Where are the quarks and electrons; the strong, weak, and electromagnetic interactions; the slight asymmetry between matter and antimatter that fills our universe with protons but keeps antiprotons out? What is missing, so far, is a precise account of how the ten-dimensional superstring, the presumed basis of a theory of everything, reproduces the world we see around us.

The superstring theory is ten-dimensional but must, of course,be brought down to four dimensions before we can make use ofit. Six dimensions therefore have to be rolled up into a small,compact, concealed geometry. We are getting used to this, and it comes as no surprise to learn that there are ways of doing it. But There are lots of ways of doing it. There is nothing in any part of string theory, so far as anyone knows, that makes a four-dimensional world special: the superstring itself is quite happy living in ten dimensions, and left to its own devices would stay there; it can be "compactified''—that is, six of its dimensions can be rolled up to yield an apparently four-dimensional world—but it could just as easily be compactified to make a three-dimensional world,or an eight-dimensional world. Superstring theory does not naturally make a four-dimensional world; physicists have to compel it to do so. And even after so compelling it, they find that there are tens of thousands of ways of rolling up six dimensions, with nothing to suggest one way over another. Here again, so far as we know, physicists have to do the choosing.

Next problem: superstring theory contains, in the form of string vibrations, lots of particles, which will eventually be identified with the quarks and the gluons, the neutrinos, the electron and muon, the photon and W and Z and Higgs bosons, and so on. But All the superstring particles are, in their pristine form, massless.We know how to give mass to massless particles, of course; that is what the Higgs mechanism is for. So mass can be provided where it is needed, and used to break the supersymmetry of the supe string so that fermions and bosons do not all come out in identical pairs. Then the Higgs mechanism must be used again, to break the symmetry between the strong and the electroweak interactions,and again to break the symmetry between the weak and electromagnetic interactions. Finally, there will emerge the world we want, the world we happen to find ourselves in.

Physicists, in a general way, know how to do all this. Theoretical progress in particle physics during the second half of this cen tury has consisted in spotting partial or broken symmetries in the interactions studied at accelerators, creating theories that embody the symmetry perfectly, and then modifying the theory so that the perfect symmetry is concealed and only a remnant of it remains for us to see. This method has given us the quark theory for the internal structure of the baryons and mesons, the electroweak theory for the unification of the weak and electromagnetic inter actions, and perhaps grand unification too. String theory is this idea taken to perfection: in the ten-dimensional world of the superstring, all particles are massless and all interactions are equal. But we do not live in that world. To get our mundane habi tation, the perfection must be thoroughly chopped to pieces and buried in a mass of symmetry breakings. The ten dimensions must be reduced to four, the particles must be given masses, the interactions must be split apart and made different. Any uniqueness or perfection possessed by string theory exists, in truth, only in that prelapsarian ten-dimensional world. Everything that must be

4one to get from there to here, from ten-dimensional perfect symmetry to four-dimensional confusion, must be done by the physicists. The superstring theory itself is silent on that issue; it does not say how it should be broken up and disguised to meet your particular needs. Superstring theory may be a perfect, unique creation, a singular piece of mathematics. But it contains, appar ently, the makings of a million different imperfect worlds, all equally possible. So far, physicists have no idea what specific ornamentation must be added to superstring theory to create the real world, let alone why that ornamentation rather than some other is preferred by nature.

From end of physics by Lindley

See also:

  • Not Even Wrong by Woit
  • The Trouble With Physics by Smolin