Hodge Dual

Intuitive

The Hodge dual, also known as the Hodge star operator ($\star$), in $n$-dimensional space takes an antisymmetric rank-$p$ tensor and maps it to an antisymmetric rank-($n-p$) tensor. If we picture the rank-$p$ tensor as a $p$-dimensional volume element, the Hodge dual can be pictured as its orthogonal complement.

Example #1: In Euclidean 3D space ($n=3$), the Hodge dual of the antisymmetric rank-2 tensor $a \wedge b$ is a vector, thus mapping rank $p=2$ to $p=3-2=1$. In this case, the resulting vector is just the ordinary cross product: $\star (a \wedge b) = a \times b$. (For the meaning of $a \wedge b$, see Exterior Product.)

Example #2: In Euclidean 3D space ($n=3$), the Hodge dual of the antisymmetric rank-3 tensor $a \wedge b \wedge c$ is a scalar, thus mapping rank $p=3$ to $p=3-3=0$. In this case, the resulting scalar is just the scalar triple product: $\star (a \wedge b \wedge c) = a \times b \cdot c$.

Example #3: In 4D space ($n=4$), the Hodge dual of an antisymmetric rank-2 tensor is again an antisymmetric rank-2 tensor ($p=4-2=2$). This is the reason why self-dual ($\star T=T$) and anti-self-dual ($\star T = -T$) tensors exist in 4D.

Concrete

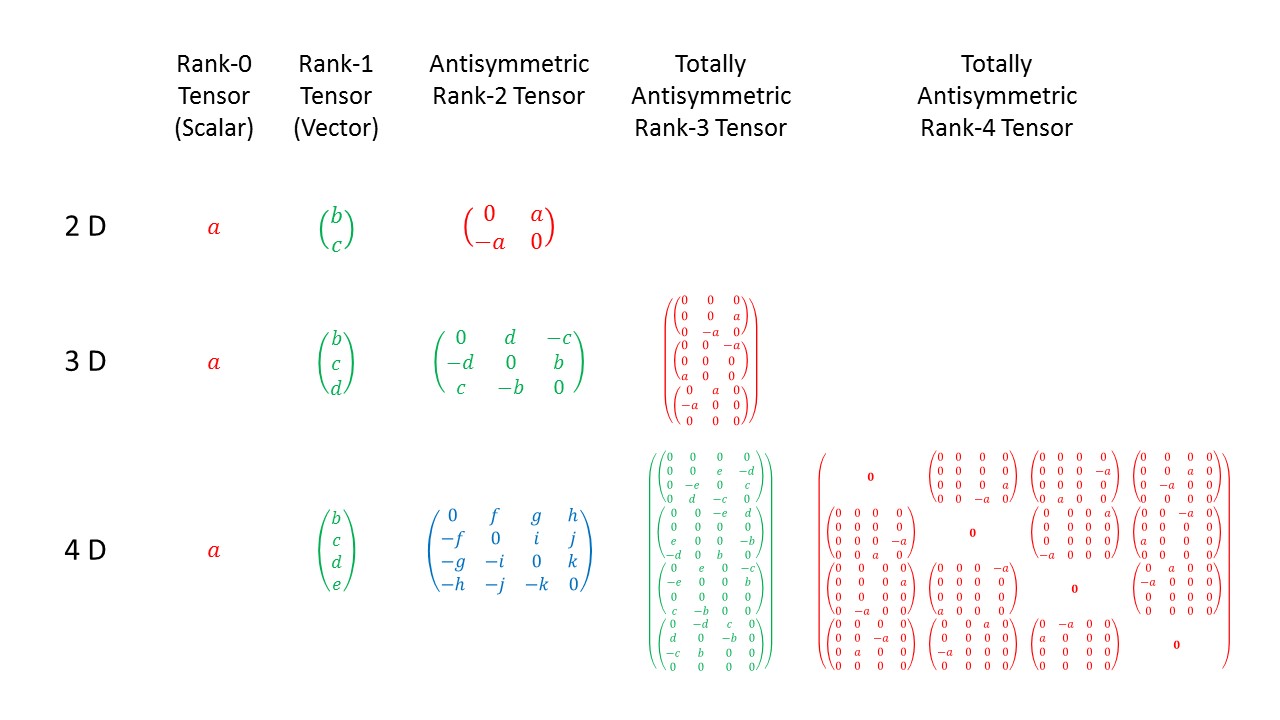
If we increase the rank of a general tensor it acquires more and more components. For example in 4D space a rank-0 tensor (= scalar) has 1 component, a rank-1 tensor (= vector) has 4 components, a rank-2 tensor has 4x4=16 components, a rank-3 tensor has 4x4x4=64 components, and a rank-4 tensor has 4x4x4x4=256 components.

The situation changes completely if we consider (totally) antisymmetric tensors instead of general tensors. Now the number of independent components increases at first but then decreases again. For example in 4D space a rank-0 tensor has 1 component, a rank-1 tensor has 4 components, a rank-2 tensor has 6 components, a rank-3 tensor has 4 components, and a rank-4 tensor has just 1 component. Notice the pattern: 1 4 6 4 1.

This pattern suggests a relationship between antisymmetric rank-$p$ tensors and and antisymmetric rank-($4-p$) tensors in 4D space. This is the idea behind the Hodge dual! The diagram below shows this pattern for 2, 3, and 4-dimensional spaces.

For a more detailed explanation of this picture see Fun with Symmetry.

Abstract

Why is it interesting?