Geometric Phase
Intuitive
When a system moves once around a close loop, like for example a pendulum once around its suspension, we expect that it returns to exactly the same state that it started in.
However, this is not always the case. System can pick up a pick up a phase while moving once around a closed loop.
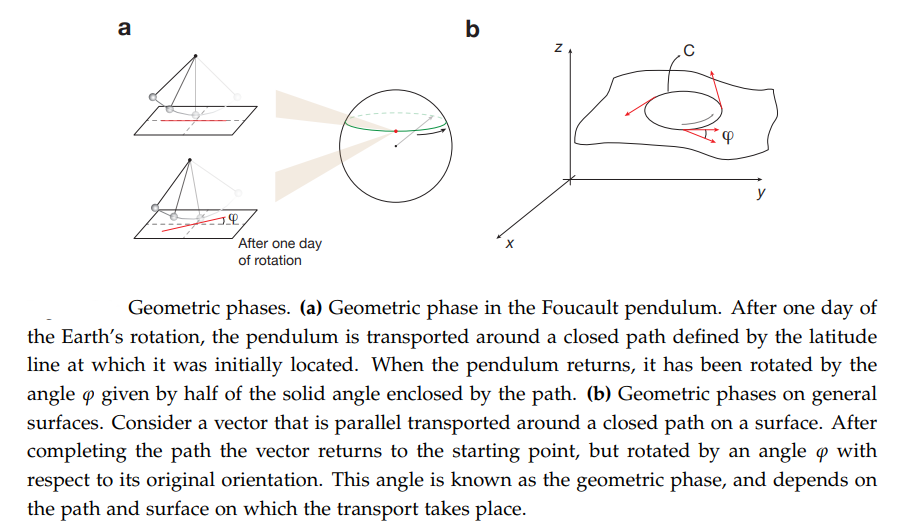
A famous example is a Foucault pendulum. Such a pendulum is expected to return to its original position after a full rotation of the earth in 24 hrs. However, it doesn’t. It picks up an angle, called Hannay’s angle.
- A nice laymen explanation of Berry phases is If You Walk in A Closed Loop, Do You End Up Where You Started? by Anshul Kogar
- Another great non-technical introduction is "The Geometric Phase" by Michael Berry.
Concrete
Before Berry reinterpreted geometric phases it was already known that if the parameters of quantum system change slowly the state of the system stays the same but it picks up a phase. This is known as adiabatic theorem. However, people believed that this phase was of no physical significance since it can have any arbitrary value.
Berry suggested that the parameters should be varied in such a way that they end up at the values they started with. The phase that a system picks up after performing such a loop in parameter space is now called Berry's phase. This phase that the system picks up after a full cycle is non-arbitrary and has profound physical implications.
- For a nice introduction, see Quantum Phases And Angles by R. Jackiw
- A nice textbook that discusses Berry's phase is Griffith "Introduction to Quantum Mechanics", especially chapter 10.
- Geometric phases also exist in classical mechanics, like for example Hannay's angle. For a discussion of Hannay's angle, see Section 4.6.3 here and also Geometric phases in classical mechanics by Morten Ib Munk-Nielsen
- The standard reference is "Geometric Phases in Physics" edited by Alfred Shapere and Frank Wilczek
- A great discussion of the Hannay angle can be found in Spivak's Physics for Mathematicians.
Abstract
Why is it interesting?
Berry phases are, in some sense, the common origin of all anomaly effects.
This is stated, for example in Berry's phase, commutators, and the Dirac sea by Michael Stone and William E. Goff